The floor

I had to tile the floor of a room which was to 2 meters long and 2 meters wide. I had boards of 20 cm wide and either 1.60 or 1.80 meter long. How many boards do I have to saw so that I can cover the entire floor?

You can check your solutions here

The 4 cards

Before you are four cards on the table. The front side has an ‘A’ or a ‘B’ on it. The back has a ‘1’ or a ‘2’ on it. As you can see, two cards show their front side, and the other two cards show their back side.
A friend of mine thinks that on the back of every card with a ‘B’ there is a ‘2’.
Which card(s) do you turn to test his hypothesis?

This is not an original problem, and the source is unknown to me. I guess it is from somewhere in the twentieth century. I was recently reminded of it when thumbing through James Fixx “More games for the superintelligent”, a mensa publication. I hope to get back to this puzzle in a later post.

You can check your solutions here

Tectonics

The free Dutch daily newspaper Metro recently – I think it was in september – published a new type of puzzle calles tectonics.
The puzzle area usually is a rectangle, for example 4×5, which is subdivided into areas of size 1 to 5. An area of size 1 contains just the number 1, an area of size 2 contains the numbers 1 and 2, and so on, until an area of size 5 which contains the numbers 1, 2, 3, 4 and 5 exactly once.
A second rule is that the same number may never be adjacent: not horizontally, not vertically, and not diagonally.
Note that there is no rule that a number may appear just once in a row or column.

A complete filled tectonic may look like:

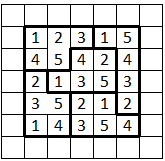
The puzzles in Metro are designed by Denksport, the largest puzzle publisher in the Netherlands. In the magazine shop I discovered a magazine with these puzzles.
Tectonic puzzle booklet can be ordered here. I think the order page is only in Dutch, and I’m not sure if you can mail order from abroad.

Nr 1)^*

Nr 2)^*

Nr 3)^**

You can check your solutions here, here, and here.

The publisher claims that these puzzles are a new international rage. That may well be true, but a quick search on “tectonic puzzles” turned up just puzzles on plate tectonics.

Digital matchsticks (4)

Move 2 matchsticks to get a correct equation:

You can check your solutions here

Bongard problems (2)

The Russian scientist M.M. Bongard published a book in 1967 that contains 100 problems. Each problem consists of 12 small boxes: six boxes on the left and six on the right. Each of the six boxes on the left conform to a certain rule. Each and every box on the right contradicts this rule. Your task, of course, is to figure out the rule.

Here is an example:

You can check your solutions here

You can find more Bongard problems at Harry Foundalis site, and I intend to publish more problems in the future.